Three Easy Ways to Transform Scary Mad Minute Drills into Something Purposeful

Yes. Learners need to know their math facts. Why? As they get older and mathematics becomes more rich and complex, working memory needs to be freed up for problem-solving. Retrieval of these facts needs to be automatic. This is just like a reader recognizing sight words as a part of literacy development. Unfortunately, curricula expectations and Common Core standards all over Canada and the United States prescribe that learners be able to “recall” or “know from memory” certain math facts by certain grades and the emphasis on timing (read: speed) and pacing becomes too heavy. 

Remember Mad Minutes? When I was in Grade 4 we had one minute to recall 50 multiplication facts. My teacher actually coached me on how to improve my speed by alternating the direction I answered the questions in so I wouldn’t waste time resetting my arm from right to left! While I did commit my facts to memory that year, it wasn’t until I started teaching and tutoring that I realized how problematic drills like these are and how they reinforce the narrative that fast = best instead of the importance of fact fluency.

In his 2015 TEDTalk on mastery, Sal Khan uses a great analogy to highlight the importance of self-paced mastery in learning. He describes how silly it would be to build the first and second floors of a home if the foundation is still wet and not to code and then argues that it is equally detrimental when we as educators “artificially constrain” the time learners have to master something – especially when we feel pressure to (or are mandated to) move on before they are ready. 

Given the tumultuous landscape that education has been (come on, all life has been) since the COVID-19 pandemic, and with many people going on about learning loss, I wish we could let go of our grip on timing. I know, I know… easier said than done and the district/administrator/state tests are coming for you by the end of the next school year. But this brings me to my main point about drilling math facts if you are going to drill at all: timing should not be the end goal. When it comes to memorization, it’s not about when, but how (Fosnot & Dolk, 2001). 

Disclaimer: you shouldn’t be thinking about drills or rote practice with math facts unless you have already been teaching for understanding. 

That being said, if you are looking for some simple repetitive practice, here are three simple shifts you can make to modify those scary mad minute drills into something more purposeful:

DRILL SELECTIVELY; NOT EVERYTHING ALL AT ONCE

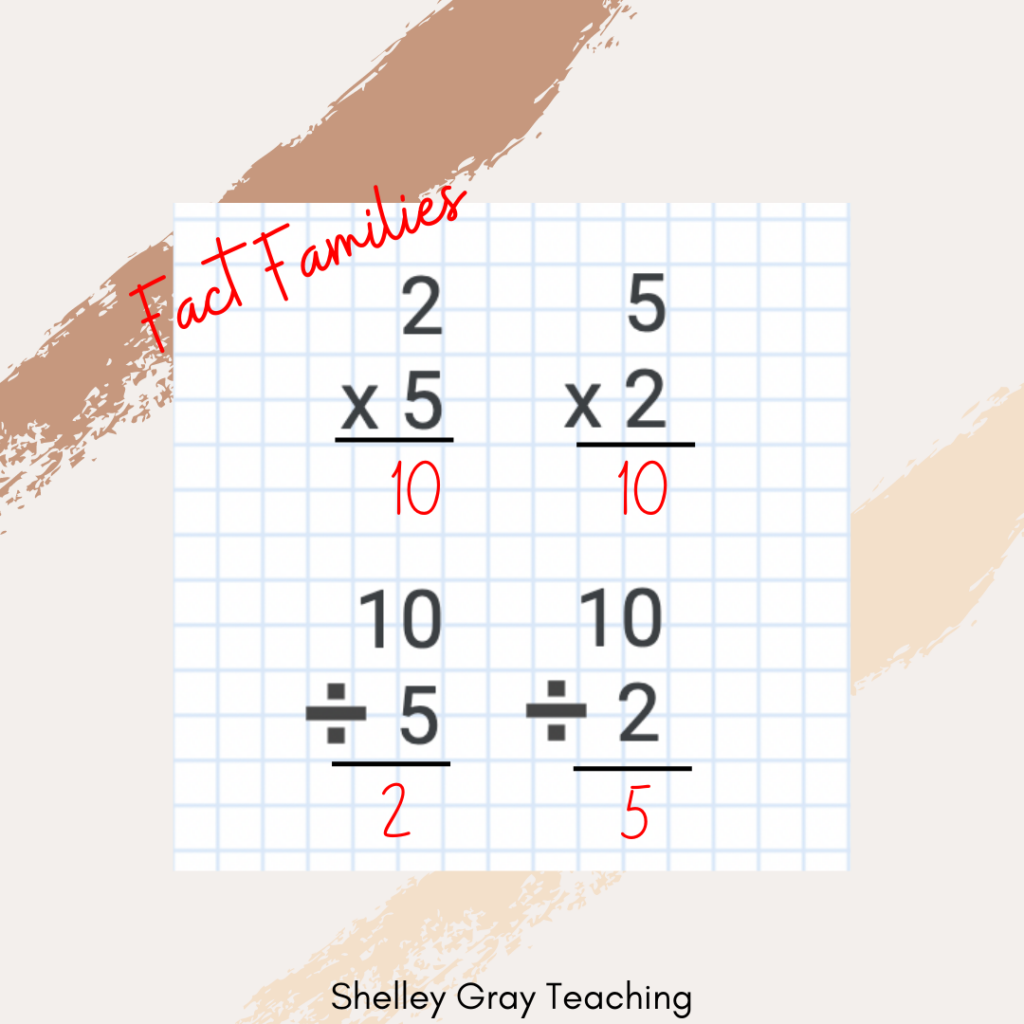
By grouping and sequencing instruction and practice around small clusters of related facts, rules, or properties, we create more opportunities for learners to make connections between operations and see patterns and relationships between numbers. An example of this would be a drill solely including questions related to multiples of 2, 5, and 10. 

BASE ACCURACY ON THE ATTEMPTED QUESTIONS AND USE A STOPWATCH, NOT A TIMER

If the learner attempts 30 out of 50 questions, score their drill out of 30 not 50. Also, have the clock run up and not down. If they answered 17/30 correctly in 6 minutes and 28 seconds, co-create a SMART goal for the next drill that focuses on accuracy first. When they can correctly answer all of the questions, then set a goal to reduce the time. Celebrate progress, personal bests and let the learner record their score.

USE ERROR FEEDBACK TO INCREASE FLUENCY

Look at the questions that the learner answered incorrectly or didn’t attempt. Look for instructional opportunities. With the clock turned off, review appropriate strategies they could use next time and bring it back to sturdy conceptual understanding using concrete manipulatives and visualization of special relationships. 

So, to drill or not to drill? How do you boost fluency and work towards automaticity in your classroom?

References

Cholmsky, P. (2011). From acquisition to automaticity: The Reflex solution for math fact mastery. Retrieved July 28, 2022 from https://www.reflexmath.com/images/Reflex_White_Paper.pdf

Fosnot, C. T. & Dolk, M. (2001). Young Mathematicians at Work: Constructing Number Sense, Addition and Subtraction. Portsmouth, NH: Heinemann.