Two Effective Ways to Make the Multiplication Facts Concrete

Are you guilty of moving too quickly through concrete models of multiplication in order to get to the abstract quicker? Most of us are. We know the end result that we want to achieve, and we sometimes skip the experiences that feel unrelated to the final goal.

The CRA (Concrete-Representational-Abstract) Model is an instructional model where we move through stages of teaching/learning. In this post we will consider this model in terms of basic multiplication facts.

In the concrete stage, we work with manipulatives and objects in order to develop an understanding of what multiplication really means. For example, we might use a rekenrek to show 4 groups of 5 or similarly 4 paper plates with 5 snap cubes on each.

In the representational stage, we represent this understanding in pictures. For example, we can use pictures of a rekenrek to show 4 groups of 5, or ten frames to show it another way.

In the abstract stage, we move to numbers and equations. This is where we will write 4×5 and expect students to understand that this means 4 groups of 5. Remember that this is the final stage and should not be our first step in teaching multiplication.

MAKING MULTIPLICATION CONCRETE

The concrete stage is an ESSENTIAL piece. This is not something that we reserve only for students who need interventions. This is an experience that ALL of our students will benefit from. Why? Because the concrete stage is where number sense is built and connections are made. Connections are often the missing piece for our students (think of the student who knows what 5×5 is but doesn’t know how to use that to figure out 6×5).

I encourage you to allow your students the opportunity to spend a lot of time in the concrete stage. This time will strengthen their conceptual understanding of multiplication. Later when you focus on the facts, this time will pay off.

Here are two important ways to make multiplication concrete.

1. Ten frames

To use ten frames as a concrete experience, we need to ensure that students are able to manipulate and work with their hands. This set of magnetic ten frame manipulatives works well to build concrete understanding. Notice that here we are showing 2 groups of 5. Because students have used ten frames in previous grades, they know just by glancing at it that there are two groups of 5. In this stage we’ll try to move students from additive reasoning (5+5=10) to multiplicative reasoning (2 groups of 5 makes 10). For an inexpensive option, use masking tape to make a ten frame on a table or the floor, and bingo chips as the objects.

Later when we begin working with ten frames in the representational stage (as shown below), students will make connections  based on how they used the hands-on ten frames. The subitizing cards below show two different representations of 4 groups of 5. These work great as “quick looks” where we show the cards for only a second or two and expect students to use strategies to figure out how many dots, rather than counting.

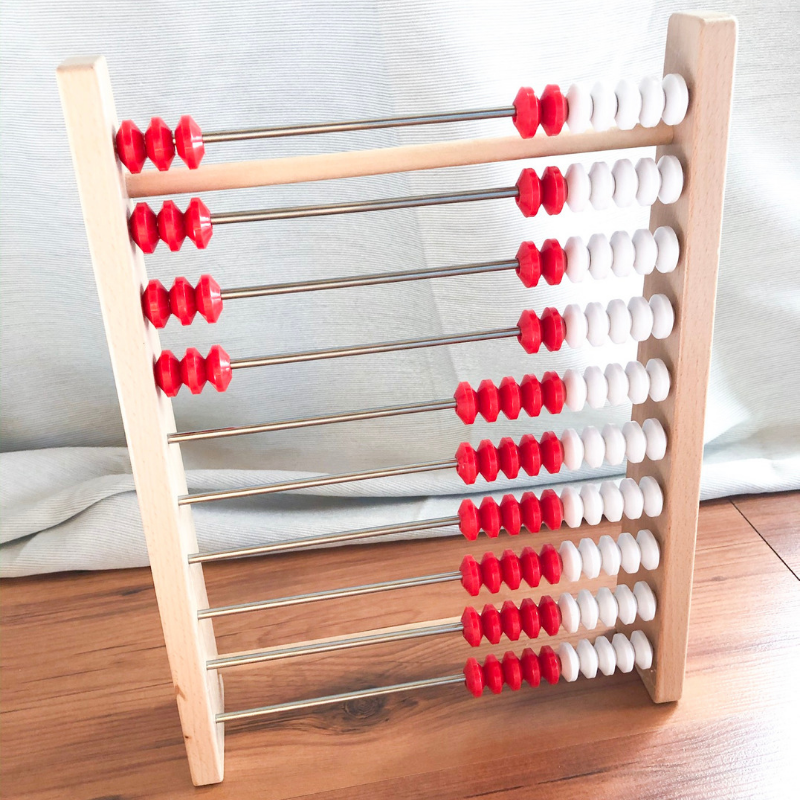
2. Rekenreks

When I was in the classroom, I had a few rekenreks that sat on my shelf collecting dust, because I really did not know how to use them properly. Ahhhh! If I would have only known then what I know now! Rekenreks are an AMAZING tool for building number sense! When you use a rekenrek, keep the white to the right side, and move beads over to the left side as needed. For example, this shows 4 groups of 3.

Notice anything with this close-up? Using a rekenrek gives your students their first experiences with arrays. This is going to enable them to make connections later on! Connections are a primary goal and are the missing puzzle piece for many students.

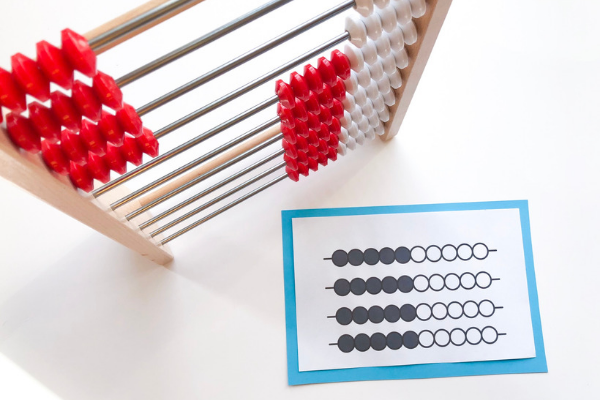
Later when we begin making multiplication representational, we can represent concrete rekenrek models in pictures. Again, notice the array in the representational picture. The card shown in the picture below can also be used as a “quick look” subitizing activity.

Fancy or expensive manipulatives are not essential to build understanding. You can easily use paper plates and small blocks to create concrete multiplication experiences.

BIG IDEA

When we give students lots of experiences with concrete and representational models, they will build an excellent conceptual understanding of multiplication as equal groups. This conceptual understanding is what will enable them to make essential connections later on. We do not need to introduce the “times” symbol until an excellent conceptual understanding has been established. This can be reserved for the abstract stage.

KEEP IT CONCRETE

Often when we do begin with concrete experiences, we tend to move on from them very quickly. Remember that even when you move your students on to the other stages, they can still benefit from being offered concrete experiences. When we can incorporate all three stages (concrete, representational, and abstract) into our teaching, our students get to see multiplication at the stage they are at. The more ways that we can assist our students in making their own connections, the better.

HELPFUL LINKS

Here are the links to the resources shown in this blog post. For full transparency, the Amazon links are affiliate links, meaning that if you order using this link, I will receive a very small commission. The subitizing cards can be found in my TpT store using the link down below.

MAGNETIC TEN FRAMES

REKENREKS

SUBITIZING CARDS